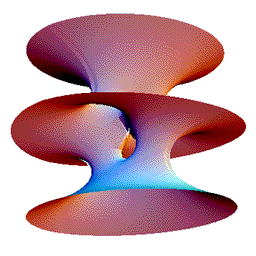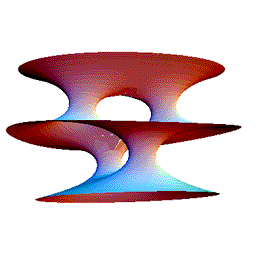The Horgan Surface

	The Horgan Surface
	The Horgan surface is a non-existing minimal surface. It looks almost as follows:

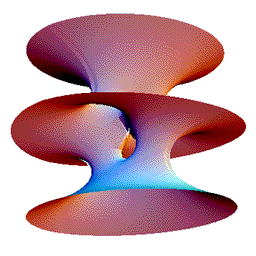	It resembles the Costa surface to the right, but the middle flat end is connected to the catenoid ends by two tunnels instead of one. However, there is no minimal surface with such properties and symmetries. Why is one nevertheless interested in it?
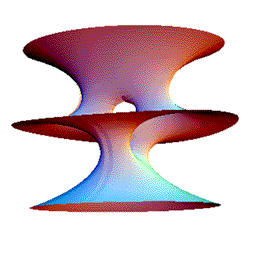
	There are (at least) two reasons. First, when first studied by David Hoffman and Hermann Karcher, numerical experiments suggested that it might exist. For this, there is one period condition to satisfy by choosing a conformal parameter, and the equation could be solved to arbitrary high precision. The surface could even be made visible. The picture below shows the remaining tiny gaps for this particular approximate solution.
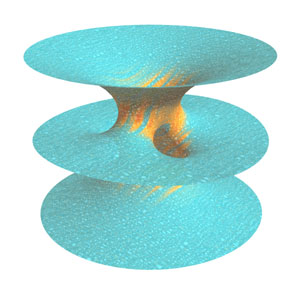
	However, with increasing precision the resulting surfaces degenerate:

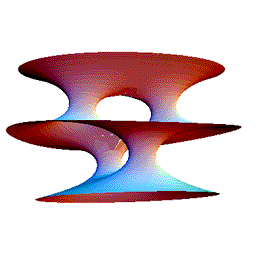	Another reason to study this surface comes from the general question how embedded minimal surfaces can look like. Recent research has produced many new examples and likely candidates, but there are also known obstructions. The Horgan surface is a simple, non-trivial example which is beyond the known general existence obstructions.
	The paper discussing this surface is here.