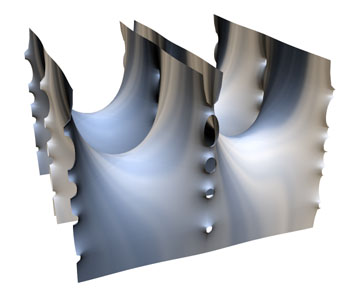
Handle Addition

	Handle Addition
	In symmetric situations one inductively can add handles to minimal surfaces. This requires the solution of arbitrarily high dimensional period problems. Due to the nonlinearity and transcendental character of the corresponding equations, this is both theoretically and numerically a very difficult problem. 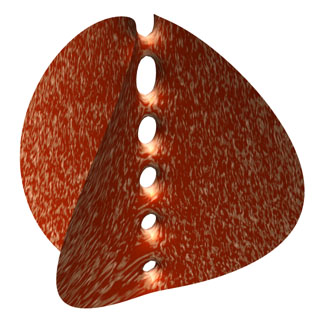Using methods from Teichmüller theory, Mike Wolf and myself have found a conceptual method for provinng the existence of all the surfaces shown here (and many more) The actual symmetry group of the surface is larger, and it is a matter of taste how large a fundamental piece one is using. The order 5 rotational symmetry of the surface is in this case very well visible.
	To the right, you see an Enneper surface with five handles. It has a single Enneper typ end. The genus 1 version of this surface was found by Chen and Gackstatter and provided the first example of a complete minimal torus of finite total curvature. The surfaces in this series have for their genus the smallest possible absolut total curvature. If the genus increases, the surfaces might converge to the singly periodic Scherk surface. A proof of this would certainly help understanding limit situations tremendously. In all known cases, limits of minimal surfaces seem to be simpler than a priori possible.
	The surface to the right was a surprise to us. We had a proof that we can add inductively in each step two handles and two planar ends to Costa's surface. The first surface in this series is a surface with five ends and genus 3 found by Meinhard Wohlgemuth. Then we realized that the same argument also allowed us just to add single handles to Wohlgemuths surface. Numerically, these surfaces are very difficult to treat, as the Weierstraß points of the underlying hyperelliptic surface are very close to each other.
	In the singly periodic situation, I was able to push the numerics further. To the right is a vertical cross section through 3 and a half fundamental pieces of a modified Callahan-Hoffman-Meeks surface: In two fundamental pieces of the Callahan-Hoffman-Meeks surface, I have added three additional handles. With increasing number of handles, a conceivable limit surface might be a singly periodic surface with a singular vertical line, found by Lopez and Martin.
	One can also add arbitrarily many handles to the doubly periodic Scherk surface. Numerically, the planar ends in between of which the handles are attached become closer and closer. If one fixes the size of the fundamental square, these surfaces might converge to two ordinary doubly periodic Scherk surfaces, shifted against each other by a half period, so that they have only vertical lines in common. This is far from provable at the moment. I also would like to know whether all these surfaces admit shear deformations.