Riemann's Minimal Surface

	Riemann's Minimal Surface
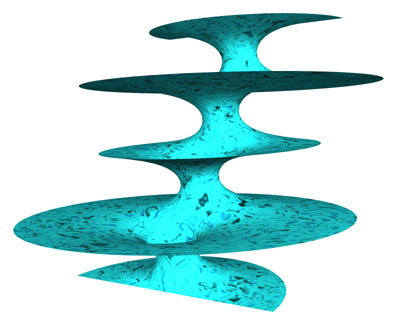
	Riemann found an interesting singly periodic minimal surface. The quotient by its translational symmetry group is a rectangular torus with two punctures representing the ends. The surface is foliated horizontally by circles which become straught lines at the heights of the planar ends.
	One remarkable property of the surface is that it has planar ends at which the Gauß map has degree two. In all known examples of embedded minimal surfaces of finite topology, the Gauß map has always degree at least 3 at planar ends.
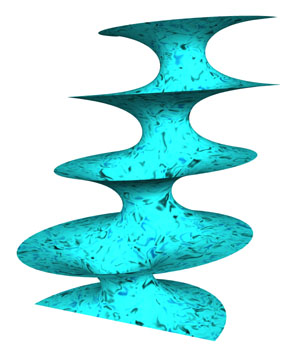
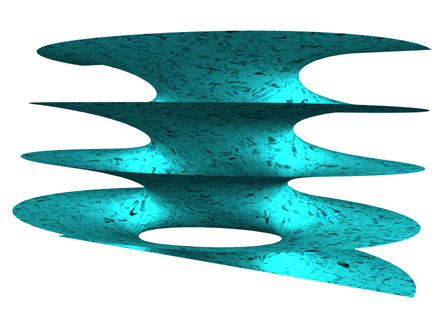
	The surface comes in fact in a family, it exists on all rectangular tori. Below are two very different cases. Under a suitable normalization, Riemann's minimla surface can degenerate to the helicoid.