Nonorientable Minimal Surfaces

	Helicoidal Minimal Surfaces, Part II
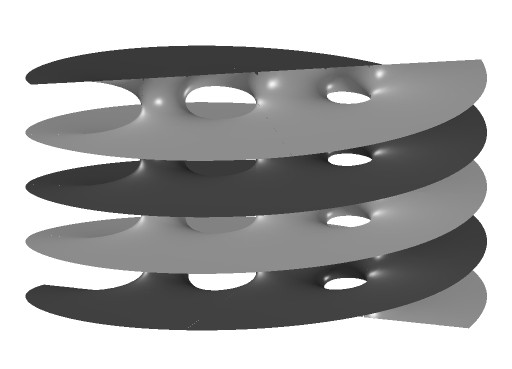
	These multigraphs are close to true minimal surfaces in the following sense: For each balanced configuration, there are 1-parameter families of screw motion invariant minimal surfaces which can be affinely scaled so that they converge to these multigraphs. Below you see two multigraphs for real configurations with charges (+-+) and (+-+-+-+-+). These surfaces are conjectured to be deformable into genus g helicoids.

	For low genus, one can produce numerical solutions to the period problem for the actual minimal surfaces To the right you can see the a minimal surface close to an affinely rescaled version of the multigraph above it. Observe that the surface to the right is slightly twisted, and the vertical lines are not straight anymore (except the middle one).
	Besides these configurations, we found another class of real balanced configurations, leading to generalized Scherk surfaces.	Below you can see again an image of the multigraph (where the points f the configuration are at -1,0, and 1, and the charges are all negative), and a minimal surface near this degeneration. Both the true minimal surface images were made with the help of Jim Hoffman, using Martin Traizet's MESH client.

	Continue

Helicoidal Minimal Surfaces, Part II