Ends of Minimal Surfaces
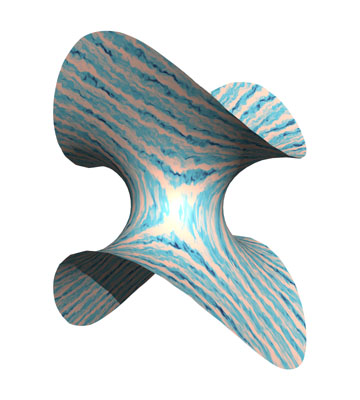
To the right you see a minimal sphere with two such Enneper ends.

Ends of Minimal Surfaces |
||
|---|---|---|
|
|
The ends of complete minimal surfaces can be as complicated as you wish. There are two reasonable assumptions which put severe restrictions on their shape: | |
| The finite total curvature condition implies that the Weierstraß data of the
minimal surface extend meromorphically to a compact Riemann surface. This means essentially
that the ends cannot be much worse then the ends of an Enneper surface. To the right you see a minimal sphere with two such Enneper ends. |
 |
|
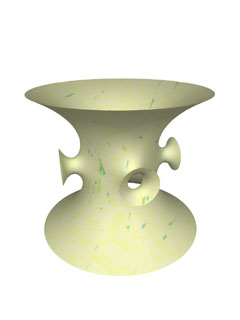
| Only two finite total curvature ends are embedded: The catenoid end and the planar end. To the right is a surface with two catenoid and one planar end. All ends are embedded but the planar end will eventually intersect the catenoid end. |  |
|
| Here you can see a sphere with six catenoid ends. There is an abundance of non-embedded finite total curvature surfaces. |  |
|
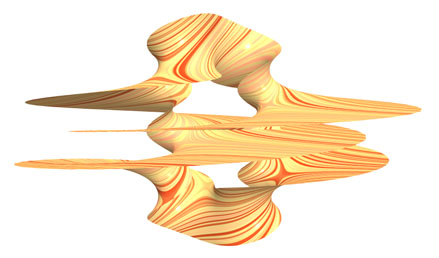
| Under the assumption that the surface is embedded and of finite topology, there are two possibilities (Collin): Either the surface has finite total curvature, or it has just one end which is asymptotic to the helicoid (Hauswirth/Perez/Romon). The latter can occur, as the genus one helicoid ilustrates. To the right you can see a piece of a minimal surface with an exotic helicoidal end. |  |
|